Aesthetic patterns for freeform architecture

About

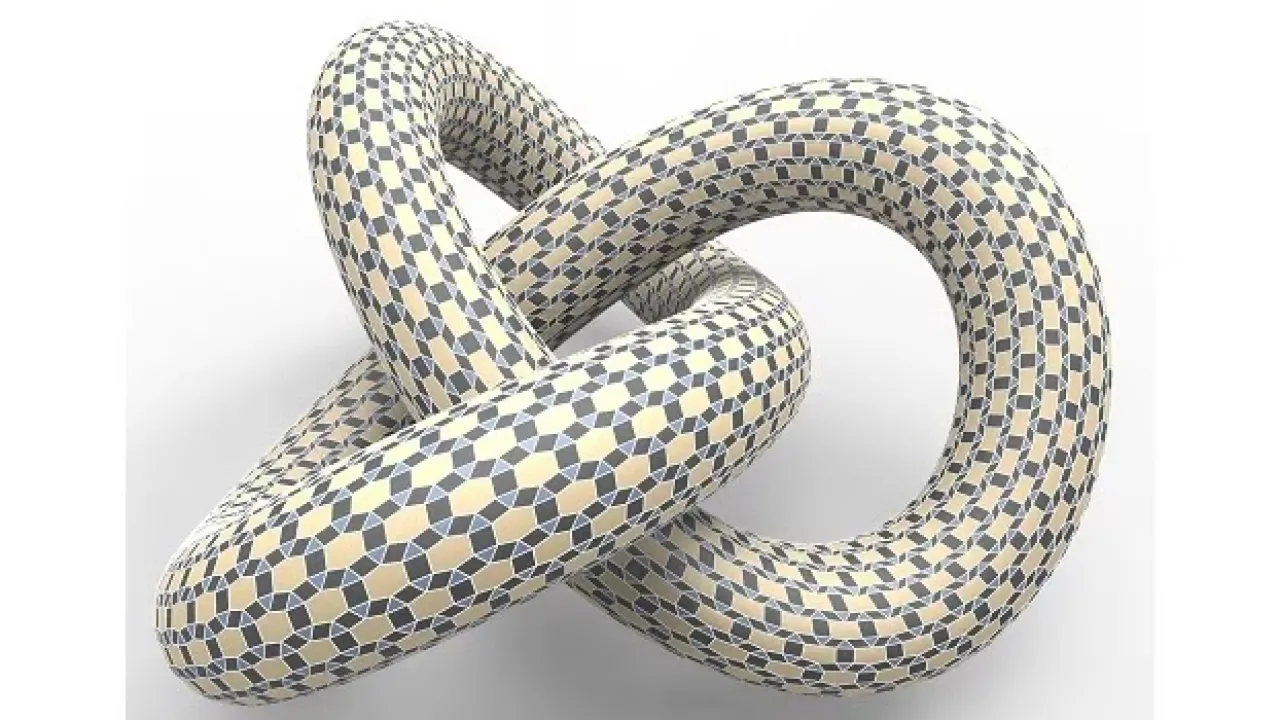
Freeform geometries are becoming increasingly common in architecture, but the construction of curved surfaces has been a long-standing constraint on such designs. Researchers from KAUST have now expanded the potential for freeform architecture by developing a computational tool that can wrap freeform surfaces in an aesthetic, regular pattern made up of different types of planar polygons.

“Renowned works like the Eiffel Tower Pavilions or the Islamic Art Department of the Louvre Museum are displays of recent progress in freeform architectural geometry,” said KAUST researcher Helmut Pottmann. “However, to keep construction cheap and efficient, a key challenge lies in creating panel layouts for such surfaces using planar panels.”

Pottmann and his team came up with a new way to lay out freeform surfaces using planar quadrilaterals, which have structural and cost advantages over the traditional triangular panels used in such constructions. However, both quadrilaterals and triangles leave much to be desired aesthetically.

“Our goal was to mix different planar polygons so that they form aesthetically pleasing patterns — a kind of interdisciplinary study of art and mathematics,” said Pottmann.

Through geometrical and mathematical analysis, Pottmann’s team found a way to generate regularized patterns of planar polygons on freeform surfaces by explicitly identifying the symmetries that are not broken by different curvatures. A symmetry-based "regularizer" algorithm then imparts a pleasing repeated symmetry on the final pattern.

“For aesthetic reasons, the patterns have to be regular in some way,” noted Pottmann. “Traditional regularities force the panels to become either concave or convex, which does not meet our requirement for planarity. Our challenge was to develop a regularizer that balances flexibility and regularity while preserving planarity.”

Read the full article