Monday, June 17, 2024, 11:15
- 12:15
https://tuwien.zoom.us/j/63202600036?pwd=sZb0q2fyAjqIUayaYM3z7J5VOfxA5v.1
Contact Person
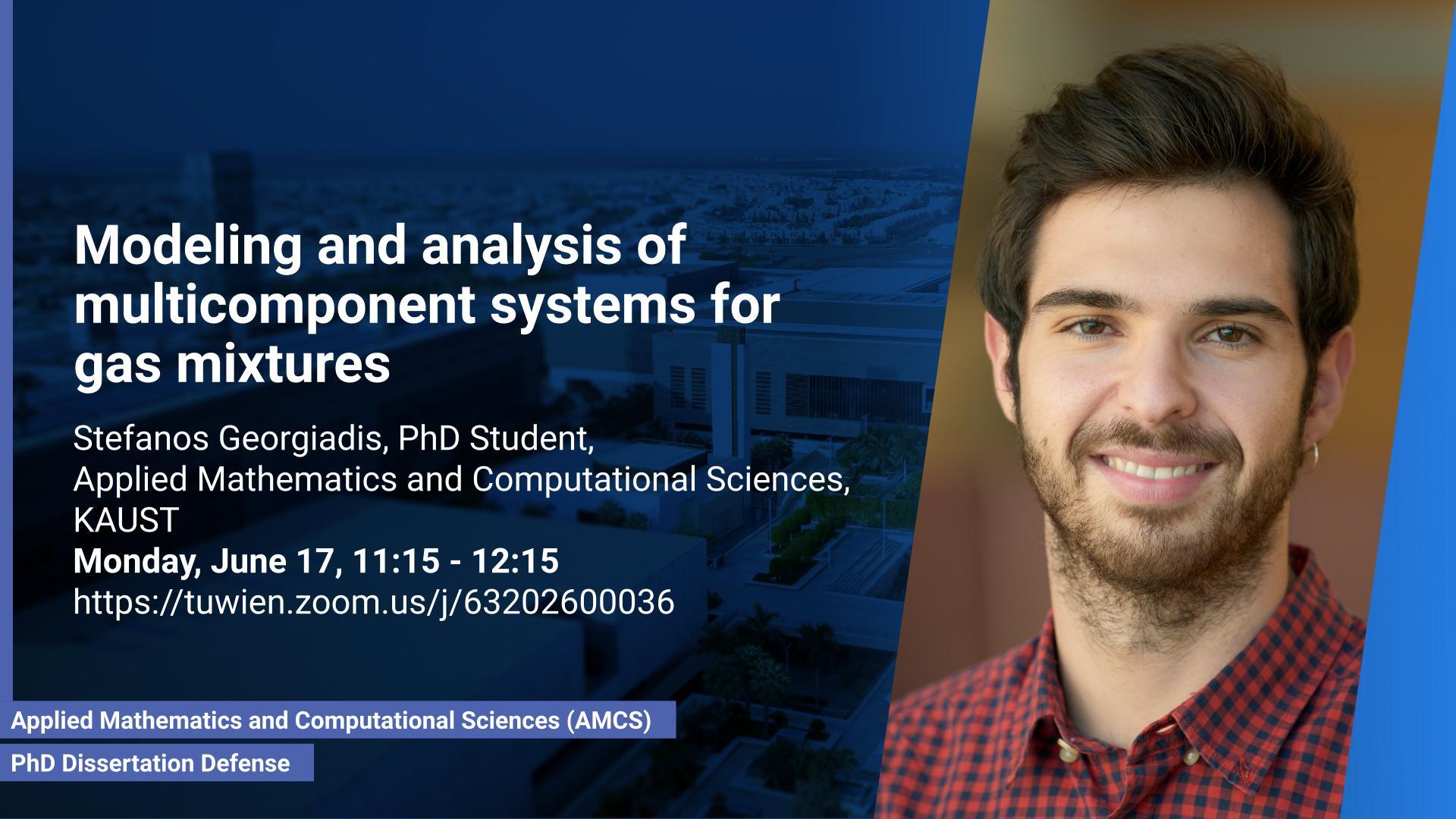
The aim of this thesis is to understand and analyze diffusive and thermal effects in multicomponent systems for gas mixtures through the perspective of partial differential equations. Starting from Class--II models of thermodynamics, diffusion equations are derived formally by a Chapman--Enskog expansion and the expansion is justified as a relaxation limit by means of the relative entropy method.